

By applying this,īy applying the property logₐ a m = m logₐ a,
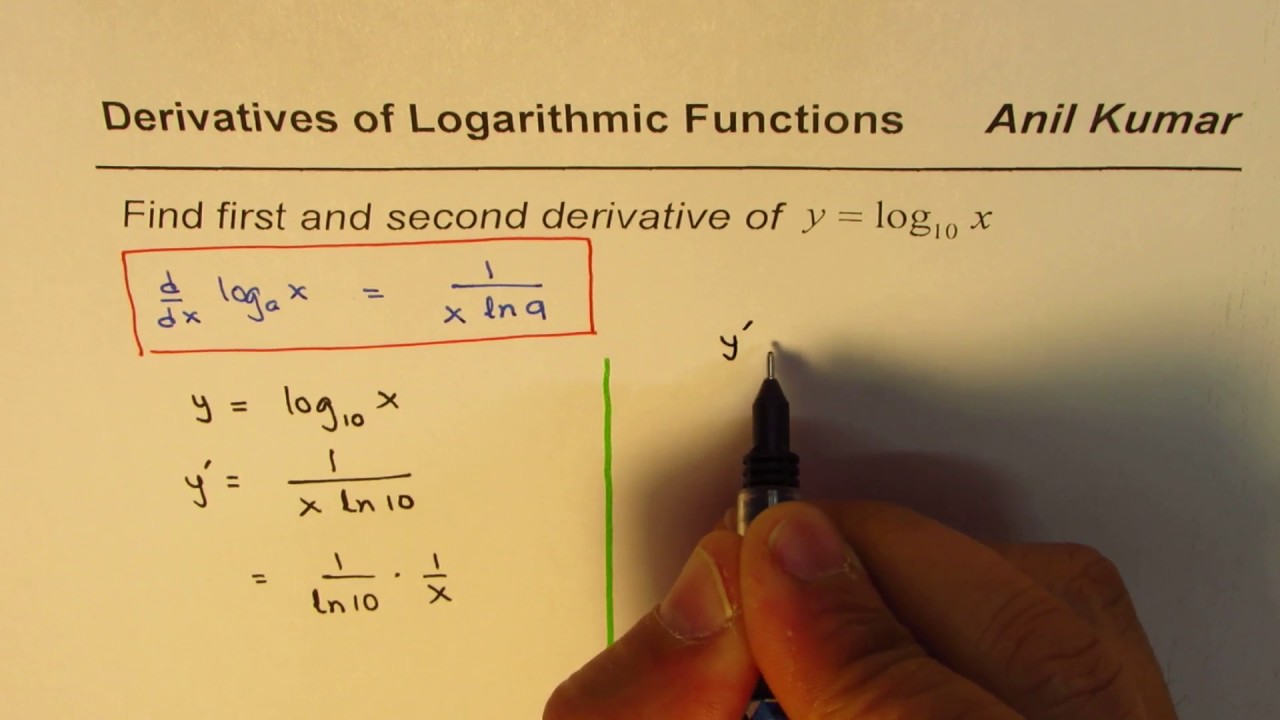
By applying this,īy using a property of exponents, a mn = (a m) n. By applying this,īy using property of logarithm, m logₐ a = logₐ a m. Using a property of logarithms, logₐ m - logₐ n = logₐ (m/n). Substituting these values in the equation of first principle,į'(x) = limₕ→₀ / h Since f(x) = logₐ x, we have f(x + h) = logₐ (x + h). We will prove that d/dx(logₐ x) = 1/(x ln a) using the first principle (definition of the derivative).īy first principle, the derivative of a function f(x) (which is denoted by f'(x)) is given by the limit, If we differentiate 1/x we get an answer of (-1/x 2).Derivative of log x Proof by First Principle

So to find the second derivative of ln(3x), we just need to differentiate 1/x To calculate the second derivative of a function, you just differentiate the first derivative.įrom above, we found that the first derivative of ln(3x) = 1/x. Ln3 is a constant, the derivative of a constant is 0
#Find derivative of log how to
How to find the derivative of ln(3x) using the product property of logs f(x) Since 3x is the product of 3 and x, we can use the product properties of logs to rewrite ln(3x): In other words taking the log of a product is equal to the summing the logs of each term of the product. The product property of logs states that ln(xy) = ln(x) + ln(y). Since ln is the natural logarithm, the usual properties of logs apply. (Regardless of the value of the constant, the derivative of ln(ax) is always 1/x)įinding the derivative of ln(3x) using log properties It’s possible to generalize the derivative of expressions in the form ln(ax) (where a is a constant value): Just be aware that not all of the forms below are mathematically correct.

Using the chain rule, we find that the derivative of ln(3x) is 1/xįinally, just a note on syntax and notation: ln(3x) is sometimes written in the forms below (with the derivative as per the calculations above). (The derivative of ln(3x) with respect to 3x is (1/3x)) How to find the derivative of ln(3x) using the Chain Rule: F'(x) We will use this fact as part of the chain rule to find the derivative of ln(3x) with respect to x. In a similar way, the derivative of ln(3x) with respect to 3x is (1/3x). The derivative of ln(s) with respect to s is (1/s) The derivative of ln(x) with respect to x is (1/x) But before we do that, just a quick recap on the derivative of the natural logarithm. Now we can just plug f(x) and g(x) into the chain rule. Then the derivative of F(x) is F'(x) = f’(g(x)).g’(x) We can find the derivative of ln(3x) (F'(x)) by making use of the chain rule.įor two differentiable functions f(x) and g(x) Let’s define this composite function as F(x): So if the function f(x) = ln(x) and the function g(x) = 3x, then the function ln(3x) can be written as a composite function. Let’s call the function in the argument g(x), which means: Ln(3x) is in the form of the standard natural log function ln(x), except it does not have x as an argument, instead it has another function of x (3x). Using the chain rule to find the derivative of ln(3x) To perform the differentiation, the chain rule says we must differentiate the expression as if it were just in terms of x as long as we then multiply that result by the derivative of what the expression was actually in terms of (in this case the derivative of 3x). This means the chain rule will allow us to perform the differentiation of the function ln(3x).


 0 kommentar(er)
0 kommentar(er)
